Multidimensional Dynamic Systems Analysis Theory

An Introduction

Lai Baoquan
Dalian University of Technology, CHN

Deng Guishi
Dalian University of Technology, CHN

Introduction

Complexity analysis is a dynamic concept in the field of systems analysis that can be traced back thousands of years. Owing to many natural and social phenomena that were not fully understood, many people became interested in research in this field. For example, in ancient China the appearance of the Book of Changes and the emergence of different philosophers and authors, particularly in the time of the warring kingdoms, reflected this research focus. In ancient Egypt people possessed a self-viewpoint on cosmic structure (Mason, 1962). In ancient Greece the elements theory of materials had been advanced (ibid.). At the same time, Buddhism gave rise to new concepts of systems thinking in ancient India. All of these were concrete examples of complexity research at the time. However, owing to limitations of knowledge and technical capacities, complexity research was then at an elementary stage. It is clear that the relationships among objects were mainly identified using subjective thinking and verified by way of thought experiments. Scientific experiments were also undertaken, such as the study of the structure of the human body in the Yellow Emperor's Internal Canon of Medicine, as well as biological research in ancient Greece. During this particular period, two completely different approaches to complexity research emerged in the Orient and the West.

Different philosophers and authors in ancient China, particularly in the time of the warring kingdoms, advanced the field of complexity analysis and promoted the development of science and technology. For example, the theory of sphere heavens, which was put forward by Zhang Heng during the Han Dynasty, contributed to the development of systems thought and methods in astronomy. From the Han Dynasty to the Song Dynasty, it could be said that the thinking of systems analysis was an important aspect of the development of science and technology. However, following the Song Dynasty, the inhibitory policies promulgated by governments in the Yuan, Ming, and Qing Dynasties and the popularizing of Zhu Xi's Confucianism resulted in the emergence of a mechanical Weltanschauung. Consequently, the energetic and dialectical systems analysis thought and methods that had characterized the previous dynasties were given less credence and deprived of the right of existence.

Regardless of these changes in philosophy, the development of science and technology in medieval China was based on aspects of complexity systems analysis and corresponding philosophical thought that had a significant impact on the development of the society. At the same time, the concept of systems analysis in the West was lost due to the effects of strict religious doctrine and conservative governments. Western civilization lagged far behind the Orient except for certain minor inventions. In spite of this situation, developments in astronomy and mathematics in the West still reflected the theoretical composition of systems analysis, particularly through the appearance of calculus and Newton's law of gravity. It could be said that complexity analysis in both the Orient and the West during this period correspondingly inherited and suppressed earlier theory and methods, and as a result complexity research stagnated.

In the first half of the nineteenth century, many achievements in the fields of natural science raised the ability of humans to understand the mutual relationships among natural events. Furthermore, the rise of philosophical reform, the development of the dialectic of Hegelianism, the further development of logical theory, and the growth of materialism thinking resulted in the rise of modern science and increased the understanding of the relationships among system components and the development of the foundation of contemporary systems analysis.

In the twentieth century, Ludwig von Bertalanffy, the founder of systematology, put forward the concept of the “system” for the first time (von Bertalanffy, 1968). This meant that the viewpoints and methods of systems analysis were abstracted from various concrete science subjects, to form modern systems analysis. Meanwhile, the field of systems analysis as an independent subject drew from and contributed to various other subjects, resulting in the mutual advancement of science. Nevertheless, along with the increasing development of science, systems analysis faced significant challenges, including the capacity of systems analysis to deal with increasing complexity; problems resulting from the growing number of dynamic variables; the nonlinear magnification of minimal disturbance; and problems resulting from rounded relationship groups (Jantsch, 1980). All of these elevated complexity research to the forefront of science research once again.

Under these circumstances, the capacity to build a reasonable theory framework of complexity analysis that addresses the above issues becomes an important factor in redefining the field of complexity analysis for the future.

BASIC ISSUES OF COMPLEXITY ANALYSIS

As far as the current state of complexity analysis is concerned, there are five fundamental issues that need to be addressed and resolved.

FADING OF DIMENSIONS

The term dimension, in brief, is being. A being constitutes a dimension in multidimensional systems analysis theory (MDSAT). Specifically, a kind of relative stability keeping fixed qualitative invariance is a dimension. On the one hand, this relative stability and fixed qualitative invariance ensure the relativity of dimensions. On the other hand, these conditions ensure the identification of the absolute attributes of the dimensions. This capacity is a special feature of MDSAT. With the formation of new matter and the expansion of current matter, dimensions increase correspondingly. On the other hand, with the disappearance and fading of old matters, the dimensions decrease correspondingly. Therefore, research regarding the increase and decrease of dimensions in the current spatial state is particularly important. The fading behavior of dimensions takes on an important role in the future development of complexity systems analysis theory, especially in determining the dimensional state extending to the endless in the “present-future” space.

SIFT OF INFORMATION

Sift of information relates to the question regarding the loss and increase of information in the course of dimensional transformation. This issue, together with the fading of dimensions, is a fundamental facet underlying future approaches to complex systems analysis. For example, in the course of dimensional transformation there is information loss resulting from the fading of dimensions. Conversely, information increase will result from the disturbance caused by the identification and the appearance of new dimensions. The resolution of this issue is a key prerequisite to the effective design and implementation of a restructured form of complex systems analysis.

FORWARD EVOLVABILITY AND BACKWARD EVOLVABILITY

Forward evolvability and backward evolvability are new concepts in complex systems analysis that must be defined. They are contracting concepts formed from the angle of metabolism of systems and their interactions with their surroundings. Forward evolvability describes such an attribute of an element collective with interactive relationships, which by adapting to its surroundings, and communicating and exchanging material, energy, and information with its environment, develops in the direction of decreasing entropy. Backward evolvability relates to an element collective with interactive relationships, which by adapting to its surroundings, and communicating and exchanging material, energy, and information with its environment, maintains its survival as its main function, thereby developing in the direction of increasing entropy.

Both of these attributes in systems reflect a course of conflict from the beginning and development of a system to the climax and death of the same system. Research and exploration about synchronous tracing and observation of both attributes will result in a clearer understanding of the features of the system, leading to a clearer, objective knowledge base for complexity analysis.

DIMENSIONAL DISTORTION STATUS IN SYSTEMS

Current systems analysis has been primarily focused on the static determination of the system structure and relationships. However, dynamic systems analysis is concerned with the twists of the system itself, the twists of system boundaries, and the dimensional twists inside systems. If adequate consideration is not given to the dimension twists inside systems, it will be difficult to understand scientifically and comprehensively the dynamic nature of relationships among elements.

BALANCE BETWEEN PROJECTING AND SUCKING OF SYSTEMS

In addition to the issues already raised, the balance between projecting and sucking energy, materials, and information from systems and their environment imposes influence on the system stability (structure) and functions. System stability is critical to the existence of a system, and also is one of the necessary prerequisites for a system to function effectively. Consequently, research on this issue should be afforded a high priority if complexity analysis is to make a significant contribution to our understanding of system structures and behaviors.

THE PHILOSOPHICAL AND LOGICAL BASIS OF MDSAT

Owing to the obvious interplay between philosophy and logic at the theoretical level, it is difficult to discuss the two concepts independently. However, in order to facilitate direct comparison of the philosophical and logical differences between MDSAT and current theories of complexity analysis, the philosophical and logical underpinnings of each approach are presented separately.

PHILOSOPHICAL CONTENT OF CURRENT COMPLEXITY ANALYSIS THEORIES

The philosophical foundation for current complexity analysis is built on three fundamental laws and various categories. The three laws are the law of unity of opposites; the law of mutual change of quality and quantity; and the law of negation.

A category is a general concept that marks divisions or coordinates in a conceptual scheme. Every subject is characterized by its own historical, special, idealistic logic measures, and these measures are required for an understanding of the attributes and natures of corresponding objects. Obviously, any science can use concepts with different open degrees and meanings, but its “skeleton” is formed by its fundamental concept categories. Categories are an integral component of the conceptual framework in any field of science. Current complexity analysis theories are mainly based on categories as follows:

• Cause and effect are categories that are common to all sciences, including complexity analysis. However, the relationship between cause and effect is often not fully understood and current complexity analysis theories do not adequately address this issue.
• Necessity and contingency are two categories that are central to complexity analysis. For example, what the edge of chaos reveals as a necessary result or contingent effect remains poorly understood from analyses of historical data. Along the historical trajectory, a significant amount of information has been filtered as a result of information sift in the evolution and transformation of dimensions. Consequently, the impact of these two categories is not completely clear at this time.
• Possibility and actuality are also categories that must be addressed by complexity analysis. A major contributor to complexity is the fact that actuality resides within possibility, while at the same time possibility is anchored in actuality. As a result, analysts are unable clearly to grasp possibility and actuality when they face complex systems.
• Content and form are categories that address the inclusive and the exclusive. Content and form are inseparable categories that both interact with and constrain each other. In analyzing complex systems, analysts should separate these but should ensure that they are not dealt with independently. Complexity systems should be understood completely on the basis that the content cannot deviate from the form and the form cannot deviate from the content.
• Essence and appearance are also essential categories for complexity analysis. The goal of complexity research is to discover the essence of complex systems, but this discovery could be hindered through interactions with appearance.
• The finite and the infinite are also necessary to complexity analysis. They will be discussed in the next section.

PHILOSOPHICAL CONTENT OF MDSAT

The finite and the infinite are two categories in dialectics. The word finite identifies matter that is compared with others, and therefore it must be taken on influence or prescription coming from others, namely it is conditional. Conversely, the infinite signifies matter that is not compared with others, and therefore it is not to be taken on influence or prescription coming from others, namely it is unconditional. As far as current complexity system analysis theories are concerned, most contents are limited in the finite and become inappropriate because of the loss of concrete limits to the infinite. It should not be said that the division of the finite and the infinite is wrong; it can be said that this division is somewhat oversimplified.

In the past, prevalent mechanistic analyses made it difficult (maybe deliberately) for people to identify the temporal-spatial distortion, and therefore it became difficult to recognize the distortion between the finite and the infinite. In fact, when the temporal-spatial distortion (distortion of dimensions) takes place, the infinite transforms to the finite in a way, while at the same time the finite transforms to the infinite. For example, in our routine life the future, the past, and the present belong to the infinite categories because they cannot be limited by conditions. But if the matter develops and changes, this infinite transforms to the finite, and this finite is different from the category of finite defined above because it is the finite in the infinite, namely it is the condition in the unconditional. As far as a period of specific time is concerned, it is finite. However, this period of time may reflect the course of infinite past and future through the interaction of the temporal-spatial distortion and matters. As a result, this phase is the infinite in the finite—specifically, it is the unconditional in the condition—and this characteristic is different from the unconditional.

Therefore, it is argued that the old categories in systems science, namely the finite and the infinite, should be developed into a new group of categories, namely the infinite, the finite of infinite, the infinite of finite, and the finite. As suggested above, this category would be in accord with the phase of cognition. The content of this category should change correspondingly at the new phase of cognition. Based on this reform, theories of systems analysis face a new change, namely that it is possible to take advantage of the infinite of finite to cognize the infinite, and to take advantage of the finite of infinite to cognize the finite. These categories are accordant with the dynamic world of dimensional (temporal-spatial) distortion.

LOGIC CONTENT OF CURRENT COMPLEXITY ANALYSIS THEORIES

Logic is a field of thought that originated in western culture. Simply defined, logic is the analysis and appraisal of arguments. From the time Aristotle created logic research, logic has always been metaphysical in its essence, following a fixed, developing direction from a definite and clear beginning to form a strict logic network. This strictness restricts normal space development so that logic is restricted in the current narrow causality space. One of the results is that many examples occur such as paradox, which cannot be solved realistically and rationally with current logic deduction and inference.

Logic belongs to the field of ontology. Along with the development of human cognition, philosophy and logic should develop simultaneously as the basis and application of philosophy. If philosophy and logic were not to develop in tandem, the development of other sciences and indeed of philosophy and logic themselves would be impeded.

Whether one subscribes to the logical system created by Aristotle or supports the system articulated by Hegel, it should be recognized that both construct logic on the basis of four-dimensional space, namely an up-down dimension, a forward-backward dimension, a left-right dimension, and a time dimension in space. In accordance with current systems analysis and logic, these four dimensions were thought to be emanative, namely they could only intersect with each other at their origin and, therefore, they could not intersect with each other at any other endless distance. However, it can be argued that there are deficiencies in this precondition: It cannot explain many actual phenomena such as abnormal temporal-spatial distortion. In this abnormal condition, the gyre of time flow may take place, namely those things that occur in the future can be shown at present. At the same time, due to spatial localization or un-transformability, this gyre takes place only on the time dimension. The result is that in a short time people completely (or at least to some extent) comprehend the scenario and/or thinking that should occur in the future.

This phenomenon, which we call foresight, is not rare and attempting to explain it based on the current four dimensions of space theory may be impossible, so it is thought to be illusion and/or mythology. However, it can be argued otherwise. If one accepts the concept that being is truth and fact, there are certain scientific theories that counteract this phenomenon. Through induction and thought, it can be argued that the four-dimensional space structure should be rectified as the basis of logic. Specifically, the concept that the four dimensions could intersect with each other on the endless distance should be explored. Only if this condition of intersection is recognized can the above phenomenon be explained.

By combining those new category groups put forward in the previous section on philosophy, it can be determined that many unintelligible and unexplainable events that occurred in the past can be scientifically explained now. For example, foreseen (rather than forecast) phenomena are, in fact, the infinite of finite. And in the infinite of finite it may be found that people in the finite time see those intended scenarios in the infinite. The infinite of finite is such a situation that the time-dimension gyres. And this gyre point is a finite entity in the infinite gyre, so people can foresee the future. Of course, as far as current cognitive theory is concerned, the thought that people can foresee the future in the absolute infinite is not supported because this kind of distortion cannot be proved in theory. Similarly, the relationship between the infinite of infinite and the finite cannot be explained clearly at present and needs to be explored in the future. (By the way, the endless discussed here is not equal to the infinite.)

Figure 1 depicts the four-dimensional structure of the current logic space.

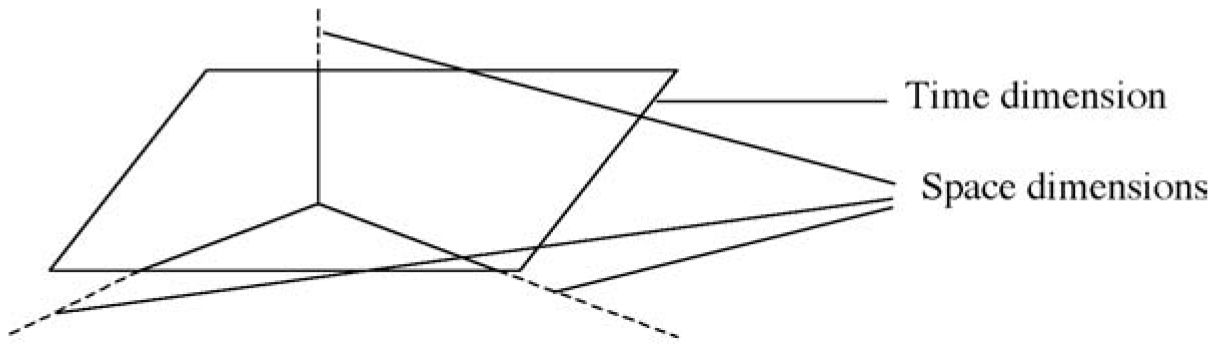
Figure 1 Current logic spaces

The principle features of the current logic system are as follows:

• Coordinate axes never intersect with each other except at the origin. Under normal conditions these coordinate axes must always be orthogonal with each other.
• The temporal axis is unidirectional. It is impossible for the future, the present, and the past to overlap or overturn (Hawking, 1998).
• There are two choices related to the above features. First, everything is controlled by God and everything in our society will evolve without any aberrations and changes according to some special plan. Second, human societies can decide on the development of society but people can only know the past and present and it is impossible for them to know the future clearly.
• Logic is based on certain premises, and inferences and deductions that include cycles during the course of logic testing are false; logic, like time, belongs to unidirectional evolution.
• The levels of division in complexity system analysis are shown with tree-level structures rather than other types.
• The philosophical categories of the finite and the infinite only include the finite and the infinite.
• Logical inference and deduction are unidirectional or bidirectional, so the corresponding trajectories should be linear. Consequently, circular trajectories mean false logical inferences and deductions.

LOGIC CONTENT OF MDSAT

Figure 2 depicts the four-dimensional structure of MDSAT.

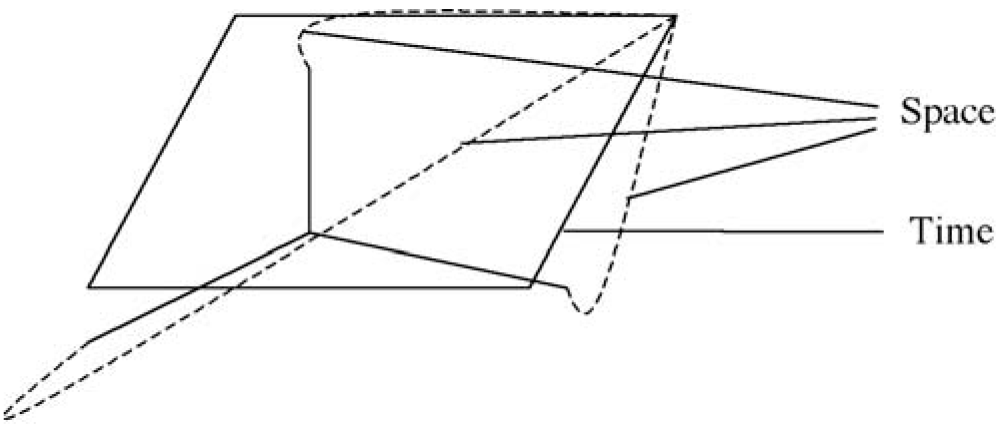
Figure 2 The four dimensions of MDSAT

In contrast to current complexity systems theory, the logic features of MDSAT are as follows:

• Coordinates can intersect with each other on the endless distance in addition to the origin. (NB: Here the endless is different from the infinite. The infinite includes the endless, but the latter does not include the former.)
• The gyre of temporal axes may take place on the condition of the finite of infinite, and also on the condition of the infinite of finite. The present reflects the future and the past. (NB: Here the gyre is different from the reversibility of temporal axes in Newtonian mechanics.)
• Development will occur through interactions of the past, the present, and the future, as shown in Figure 3.

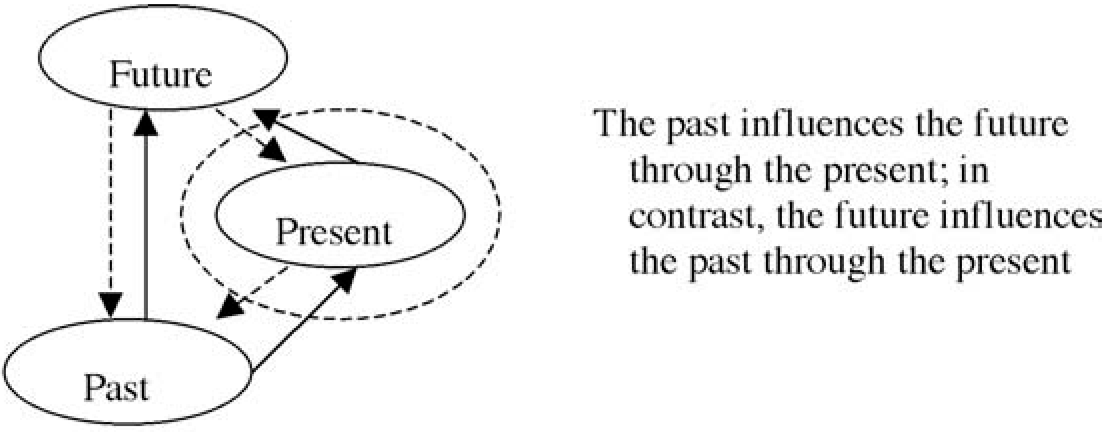
Figure 3 Relationships among the past, the present, and the future

• Cycles in the course of logical inference and deduction are important precursors to the discovery of the next phase of inference and deduction. Logic has not been purely unidirectional, and qualitative changes of logical inference and deduction are known to occur during the development of the infinite. These qualitative changes result in the iterance from simplicity to complexity and from the meaningless to common action among the past, the present, and the future. As a result, the infinite becomes the finite being, and then acts on either the present or the future.
• The levels division of complexity system analysis is not based on a tree-level structure. Rather, it can be shown as a dynamic cycle (namely a matrix form), a spatial ball, or other more open forms.
• Philosophical categories expand from couples to groups. For example, categories coupled as the finite and the infinite expand to a group to include the finite, the infinite of finite, the finite of infinite, and the infinite. This spiral up-evolution reflects an open logic structure in philosophy. Consequently, the term “open logic” can be used to describe a logical system that is constructed in four-dimensional space.
• Logical inference and deduction are three dimensions or more. Under these conditions, some logical problems that were once thought to be paradoxes can now be solved. For example, the “dollar paradox” in finance is not a paradox when viewed from the angle of inference and deduction in a multidimensional system. If the temporal dimension is added, the appearance of the strong and the weak dollar will be extended to three dimensions, rather than the two-dimensional structure in current logical inference and deduction. By applying the condition of three dimensions, a cycle of logic cannot be constructed, so the paradox does not exist. This explanation is presented schematically in Figure 4.

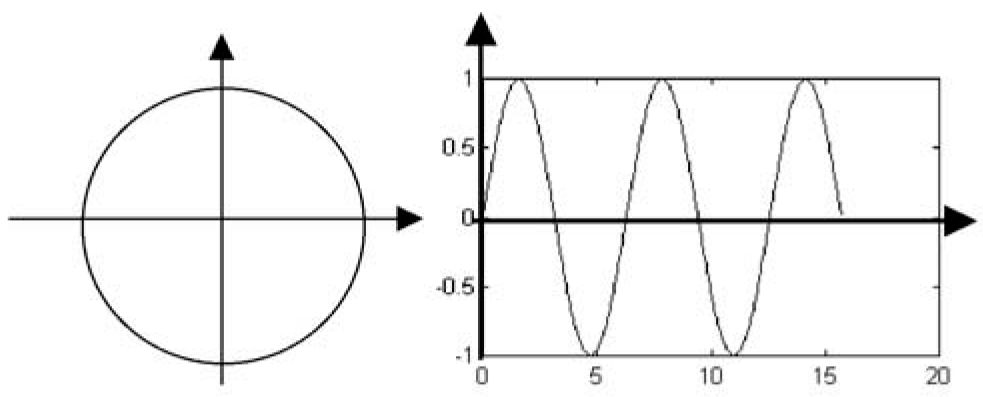
Figure 4 Two different types of logic inferences

These characteristics represent the main differences between current logic systems and the open-logic structure of multidimensional dynamic systems analysis theory. Examples of the application of open logic will be provided in subsequent sections.

MULTIDIMENSIONAL DYNAMIC SYSTEMS ANALYSIS THEORY

Simply put, MDSAT is composed of three parts: structurability resulting from dimensions; variability resulting from the acceptance of the dynamic nature of systems; and traceability of full course to complexity systems.

The core of MDSAT is to use the invariability and variability in the course of the evolution of systems to analyze complexity systems. For example, in the course of a seed sprouting, growing, becoming a mature tree, breeding, and dying, invariabilities include many facets. Specifically, the seed becomes a tree at the moment of sprouting and, whether big or small, it is a tree in nature from that point. Therefore, the tree assumes a degree of invariability, and based on this analysis the bark and trunk also are fixed. The corresponding difference only results from the different phases of growth, and these differences belong to the category of variability (dynamicability). Similarly, because of differences in environmental conditions for trees during their growth cycles, it is inevitable that differences in dynamicability will occur. Furthermore, due to the feature of uniqueness (or homo-uniqueness) that characterizes most complexity systems, finding the equilibria and laws between the invariability (structurability) and the variability (dynamicability) becomes a necessary prerequisite for effective complexity systems analysis (that is, trace in full course becomes more and more important). MDSAT is structured to achieve this objective.

MAIN CONTENT OF MDSAT

In this section seven steps are identified to facilitate the use of MDSAT in the analysis of complexity systems.

Determining systems and corresponding elements and relationships

This step is generally analogical with current systems analysis methods. However, since the object systems are more complex, the quantities of elements and relationships in systems and environmental variables that must be addressed exceed those that can be dealt with using current systems analysis theories. This step is the basis for later steps, and needs to be collaboratively completed by systems analyzers and correlative personnel.

Determining the dimensions state in systems and their surroundings and the state space during the course of tracking in the past, present, and future

This step is the principal basis of MDSAT. If it is not completed successfully, all subsequent steps will fail, and MDSAT will regress to the state of current systems analysis theory. What should be clear is that this step comprehends dynamicability through a greater understanding of those slices (analogical with the function of Poincaré slices) in each phase during the course of initiating and developing systems. Only based on finding a system's dynamicability can the corresponding dimensions, the dimensions' attributes, and the basic status of each dimension be determined (this is the so-called mapping from dynamic to structure). Furthermore, the environment outside the system's boundary should be the focus of similar investigations so that research related to input and output does not breach validity. These investigations also can ensure that there are sufficient accurate data for analyzing the projecting and sucking between the system's boundary and its environment.

Determining the dimension of the system boundary

From the point of view of systems analysis and practices, it can be determined that differences exist between a system boundary and the clear conjunction between systems and their surroundings. The system boundary usually exists with a distorted shape, and cannot be replaced with such a superficial conception as the clear conjunction between a system and its surroundings. Determining the dimension of the system boundary will result in cognition of the nature of the relationships between the dimensions of the system and those of its surroundings. At the same time, based on research related to the projecting and sucking of systems, it can be established that many projectiles and sucking materials are determined by system boundaries rather than the systems themselves. As far as systems and their surroundings are concerned, they may be normal on the surface, but system boundaries as friction straps between systems and their surroundings usually are abnormal.

Researchers generally ignore this abnormality, but it will gain more importance in the near future. As demonstrated by fractal geometry, even the boundary between land and ocean, which has been thought a simple place in the past, exhibits distortion of dimension, namely the infinite of finite space. As another example, the fractal structure of trees indicates that the structures of boundaries between trees and their surroundings exist in distorted dimensions, rather than the structures that trees as systems show in corresponding surroundings, or the structures of the internal dimensions of trees as systems.

Determining the dimensional distortions to internal elements of systems

As systems may be divided into subsystems, systems analysts also must address the relationships of dimensions between these internal subsystems and their parent systems. Systems are composed of elements and relationships, but systems analysts often know little about the dimensional transformations of these elements and relationships. This is a significant deficiency that often results in a superficial analysis of a system and a failure to comprehend the fundamental attributes of the system. From some aspects, systems are finitely divisible, namely it is possible to determine the lowest structure cell that influences the functioning of the system. By undertaking even cursory research, dimensional distortions of system elements will be simplified. Specifically, the interactions can be determined between behaviors of dimension actions and other elements and the dimensional transformation relationships between these interactions and the whole system.

However, such simplified analyses are becoming more and more inapplicable in our current complex society. In this development stage of human society, the importance of enhancing our knowledge of complex systems and the capacity to divide systems and subsystems becomes magnified more precisely. Consequently, the need to understand the impact of dimensional distortions on system behavior becomes more significant. Typically, it may be found that distortions take place to many elements in a system, and these distortions can be so serious that the whole system is completely distorted, along with its numerous subsystems (elements). With the closing from macrocosmic to microcosmic and the strengthening of flashing back from microcosmic to macrocosmic, the dimensions of systems are no longer those simplified determinations of the past. In fact, dimensions now inhabit a kind of space in which high dimensions are present/included in low dimensions, low dimensions overarch high dimensions, complexity reflects simplicity, and simplicity includes complexity. Based on this space, one can divide these dimensions infinitely. Although there may be reiterations between the macrocosmic and microcosmic in some taches, every reiteration will result in increasing innumerable (unbelievable) double complexities. Recognizing this fact, the capacity to determine dimensional distortions to internal system elements becomes a very important analytical method and step.

Determining problems related to system projecting and sucking

As pointed out in step three, system projecting and sucking are mainly determined and completed at the system boundary. In contrast to functions exhibited by internal system elements, projecting and sucking at the system boundary deal with objects different than system inputs and outputs. These differences may be a function of collapses and swells of internal dimensions in system influence elements that then act on the system boundary. In addition, the differences may be a result of the system and its surroundings grinding with each other or certain special attributes of the system and its environment.

The impacts on systems resulting from these projecting and sucking materials and their influence on relationships between systems and their surroundings remain largely unknown. However, it can be argued that projecting and sucking are special attributes of systems. It can be further argued that systems themselves are complex, so that they cannot be clearly depicted with past simplified structure models or single function analysis. Through an analysis of projectiles and sucking materials, it may be possible to determine what systems need and excrete, thereby enhancing our knowledge of the evolving direction of the system boundary and the direction of metabolism.

Based on the above, the conflict and unification between a system's elements and its boundary can be determined more precisely, which will assist researchers to grasp systematic metabolism. At the same time, through analyses that combine projecting and sucking with system input and output, it may be possible to understand more fully the relationships between system functions and structure and those between systems and their surroundings. Such analyses would clarify the main relationships resulting from system functions, the subordinate relationships resulting from projecting and sucking, together with the relationships between the two. Finally, the influence that these relationships have on the surrounding metabolism can be identified, so that complexity systems may be cognized more effectively.

Establishing dynamic models

Using analytical results from the above five steps and corresponding dynamic relationships helps to determine the relationships of system elements and corresponding dynamic changes and phase functions. Dynamic models based on MDSAT should reflect the complete dimensional structure of systems, their corresponding surroundings, all system elements, and system boundaries. Furthermore, dynamic relationship groups must be recognized, including relationships between systems and their surroundings, between systems and their internal elements, among internal elements, between internal elements and system boundaries, between system boundaries and systems, between system boundaries and their surroundings.

It is impossible using current structure models to substitute these dynamic models, but they may be expressed with several simple structure models with the time factor added. Of course, the dynamicability of relationships among all parts needs to be depicted and denoted in any new model. Therefore, it is suggested that a perfect dynamic model is at least composed of three types of models (herein the so-called XX figure is a habitual denotation, rather than a real figure): models of relationship motion figures (including all the dynamic structures inherent in the above relationships); models of element motion figures (including all the dynamic structures of the above elements and relative gestalts); and models of system motion figures (depicting the dynamic status of the system as a whole).

Operating and achieving results

Since a significant amount of data are used in MDSAT analyses, many sets of calculations are required to complete each of the above steps, and their results must be integrated. This is because the results of general systems analyses cannot be simply reflected with calculation results coming from each step. By completing the above analyses in an integrated manner rather than simply quantifying the contents in the sixth step, the results will include: when and which kind of relationship takes on the decisive role; when and which kind of element has the strongest influences on system functions; and when dimensions of systems change.

The application of these seven steps of MDSAT will establish a system analysis theory and tool that are more suitable to contemporary needs.

KNOWLEDGE RELATIVE TO MDSAT

The following concepts are critical to a full understanding of MDSAT.

Space colonizability

Space colonizability has a significant influence on hierarchy analyses and dimension analyses in MDSAT, namely elements in different space scales are usually suited to corresponding levels. As far as space colonizability is concerned, it is still systematic individual behavior, and cannot reach the category of hierarchy.

Metabolicability

Metabolicability relates to the evolvability of systems and is a critical element of MDSAT.

Regularability and inhibitability among levels

Regularability and inhibitability among levels are inter-influences between levels and, therefore, are the main foci of hierarchy analyses. In this regard, the main consideration is the regularability and inhibitability from the angle of pure levels on the precondition of leaving the relationships between the system and its surroundings.

Attractability and repellence among iso-level systems

This aspect is related primarily to the input and output behaviors of systems. However, it should not be confused with input and output analyses. Iso-level systems denote those systems in the surrounding environment that may input and output with the object system. Having input and output behaviors with iso-level systems denotes attractability, or repellency.

Influence coming from simisystems

This aspect describes influences exerted by exterior systems on the object systems through system boundaries. The fundamental preconditions to understanding this aspect are that input and output mainly counterpart with system functions; and projecting and sucking through the system boundary are mainly counterparts of the system structure.

The evolution of minimal self-analog of systems

The evolution of minimal self-analog of systems is a counterpart of systems in different stages. As systems evolve, the evolution of minimal selfanalog becomes more complex. Knowledge of this process is crucial to analyzing complexity systems with MDSAT. Even for those totally unacquainted with complexity systems, it is possible to achieve a breakthrough when analyzing them from the angle of minimal self-analog.

APPLICATION FIELDS AND ADAPTABILITY OF MDSAT

APPLICATION FIELDS

Based on the above discussion, application rules for MDSAT may be generated to support the utilization of this new type of systems analysis on complexity systems. Because static systems may be considered as special examples of dynamic systems, MDSAT may be used to analyze them and favorable effects may be achieved. Since MDSAT involves very complex analyses, collaboration among analysts from various disciplines may be required. For example, experts in the fields of mathematics and computing can contribute to the calculations of dimension distortions, as well as syntonic analyses of relationships among elements. All of the above need a group to work cooperatively, which shows the complexity of analysis subjects—namely from personal analysis to collectively cooperative analysis—coming from the complexity of object systems.

The fields of application of MDSAT are any dynamic system, or any system with a high-complexity appearance (whether they are material systems, or psychic systems, or both).

ADAPTABILITY

Only if a theory is self-consistent and effective in practical usage can it be said to be applicable. MDSAT is self-consistent, namely it has its own starting point, it possesses strong philosophical and logical foundations, and it is not self-conflicting in structure. At the same time, this theory has practical applications. For example, it may be used favorably in such fields as economic systems analysis, financial systems analysis, and other real complexity systems. The application of MDSAT in these situations will be addressed in subsequent articles.

References

Gensler, Harry J. (1989) Logic: Analyzing and Appraising Arguments, Englewood Cliffs, NJ: Prentice-Hall.

Hawking, Stephen W. (1998) A Brief History of Time: From the Big Bang to Black Holes, London: Bantam Books.

Jantsch, Erich (1980) The Self-Organizing Universe: Scientific and Human Implications of the Emerging Paradigm of Evolution, Oxford, UK: Pergamon Press.

Mason, Stephen F. (1962) A History of the Sciences, New York: Collier Books.

Perry, John & Bratman, Michael (eds) (1993) Introduction to Philosophy: Classical and Contemporary Readings, New York, UK: Oxford University Press.

von Bertalanffy, Ludwig (1968) General System Theory: Foundation, Development, Applications, New York: George Braziller.