Archetypal dynamical systems and semantic frames in vertical and horizontal emergence
William H. Sulis
McMaster University, CAN
Abstract
This paper introduces the main concepts and constructs of archetypal dynamics, a formal approach to the study of emergence based upon the analysis of coherent, meaning-laden information flows within complex systems. Two forms of emergence, horizontal and vertical, are discussed, and four dyadic modes of information, public-private and active-passive. The fundamental triad of archetypal dynamics consisting of semantic frame, realizations (system) and interpretation (agent/ user) is introduced. The formal representation, the tapestry, is defined and the notion of H-complex tapestries is introduced, along with proposed connections to horizontal emergence.
From vertical to horizontal emergence
Archetypal dynamics is a formal framework for dealing with the study of meaning laden information flows within complex systems. The main goal of archetypal dynamics is to study emergence in complex systems, but it carries sufficient descriptive and explanatory depth to serve as a foundational language for describing complex systems generally. This paper deals with the underlying philosophical concepts, introducing the basic mathematical representation, the tapestry, and provides an introduction to emergence through the idea of H-complexity.
Archetypal dynamics began with the realization that our current concept of emergence had become quite narrow, being restricted primarily to emergence from lower to higher spatio-temporal scales. Traditional examples of emergence include such situations as the formation of matter out of fundamental particles, of organisms out of cells, of species out of individuals, of minds out of neurons. These are all examples of what can be termed Vertical Emergence, as they all involve the seeming appearance of one class of phenomena, occurring at one spatio-temporal scale, out of a different class of phenomena occurring at a higher spatiotemporal scale. The phenomenon which appears at the larger spatio-temporal scale, the emergent, is said to have emerged from the phenomenon occurring at the lower scale through a process of emergence. The concept of emergence has thus been restricted to situations involving upward causation, in which high level processes are in some sense determined by or caused by lower level processes.
This bias in focus reflects the disciplinary origins of recent attempts to distinguish the notion of a complex system from its close cousin, the complicated system. A complicated system is one composed of a large number of small sub-units that are dynamically coupled to one another and whose large scale behavior can, in principle at least, be understood as a direct consequence of these low level interactions, e.g., the well over one million parts of the Saturn V rocket used to place an Apollo crew into orbit. Although in principle, the equations of motion for such systems can provide a predictive model for their behavior, they are seldom solved in practice except to ensure solutions where small perturbations in the system’s state give rise to only small deviations in behavior.
The discovery by Poincaré at the turn of the twentieth century of chaotic dynamics, and its rediscovery by mathematicians and physicists some 60-80 years later, demonstrated some fundamental limits on the practicality ofbehavioral predictions for complicated systems. In particular, there arose the awareness that large classes of systems exhibit sensitive dependence on their initial conditions, meaning that small deviations in state become amplified over time resulting in an inability to make long term predictions of behavior. In an effort to better understand the conditions under which chaotic dynamics might occur, researchers began to explore a wide range of simple models of chaotic systems, usually through computer simulations since the equations of motion for these systems are not exactly solvable. This led researchers to the discovery of systems whose large scale behavior appeared to be so far removed from that of its low level constituents as to constitute in their mind an entirely new phenomenon. These researchers arrived at a communal intuition that systems that were deemed to be complex exhibited behaviors and structures that appeared to transcend those at the lower levels of their constituents. Although not rigorously proven, this insight led these researchers to coin a new term, that of a complex system and of its attribute, complexity (Forest, 1990).
The early complex system models were themselves complicated systems, generally being composed of large numbers of smaller sub-units interacting in complicated ways. Thus there was an explicit recognition of at least two distinct spatio-temporal scales governing the descriptions and dynamics of these systems. Since the reductionist paradigm that has been so influential in the sciences, especially the hard sciences, asserts that a complete understanding of the behavior of a system at the lower spatio-temporal scale would be sufficient to enable one to predict and to describe the behavior of the system at the larger spatio-temporal scale, the discovery of dynamical chaos put a damper on the general applicability of reductionist principles, though it did not cause the majority of scientists to abandon it entirely. Although the discovery of the concept of the complex system seemed to deal a death blow to the reductionist ideal, in fact that did not happen because these researchers asked themselves whether there might exist some general principles that would determine when a system would be complex. Some headway in this regard had already happened in the study of chaotic systems, and since reductionism had been a fundamental principle for many of the researchers moving into this field, it was natural that a concerted effort would be undertaken to preserve it. With that goal in mind the Santa Fe Institute was born. In its early years a great many notions of complexity began to appear in the literature. Almost always, these notions and models involved coupling phenomena that appeared at distinct spatio-temporal scales. Most definitions focused on the issue of the loss of predictability as one moved up the spatio-temporal scales. Thus the main focus of these researchers has been on vertical emergence and the general notions of emergence that have come out of this literature are mostly limited to situations of vertical emergence.
The concept of emergence, as pointed out by Goldstein, (1999), is actually quite old, dating back to the nineteenth century, and much broader in scope. An expansion of the concept of emergence beyond that of vertical emergence yields new insights into its essential character. For example, the study of vertical emergence has focussed upon the loss of determinism imposed upon upward causation in vertically emergent complex systems. But why should one be limited solely to upward causation as a determinate principle? There are many examples in the literature of emergent downward causation. A striking example appears in neurobiology (Anderson, et al., 1995; Sulis, 2000). There is abundant evidence that certain neurons modify the dynamical response characteristics of some of their receptors in response to patterned stimulation from upstream neurons. These receptors are modified if the upstream neurons fire with a particular timing, or fire in conjunction with a background of Theta rhythm activity. Thus large scale patterns in the perceptual environment impose spatio-temporal patterns on the firing activity of sensory neurons, and these patterns in turn trigger off functional changes in other neuronal populations. Thus a pattern at a large spatio-temporal scale induces changes in neurons at a much smaller spatio-temporal scale. A more arcane example derives from Jung’s ideas about the influence of the collective unconscious on individual behavior (DeLaszlo, 1959). In Jung’s psychology, the behavior of an individual is determined not solely by influences, conscious or unconscious, that can be considered as arising within an individual, but in addition by influences that arise from large scale patterns present within particular cultures as well as from historical and evolutionary factors. The presence of downward causal influences confounds the question of which phenomenon came first, the lower or the higher, and which phenomenon actually emerged. Thus even in the case of vertical emergence, causal influences and determinate processes are none too clear.
Another rather subtle example of emergence is that of Goldstein’s (2003) “self-transcending constructions.” Here the emergent appears as a result of the system acting upon itself in a reflexive process. An example offered by Goldstein is Cantor’s Diagonalization method. Another example is the transcendental construction giving rise to Russell’s Paradox. (See Appendix I for a more complete technical discussion of both examples). Both of these examples are rather abstract, but the point is that applying an operation reflexively to some structure may give rise to a new structure that transcends the old in some fashion. Goldstein would say that the new structure has emerged out of the old by means of this self-transcending construction.
The idea of emerging ‘out of’ suggests that all of the factors required for the appearance of the emergent through the process of emergence reside within the original phenomenon. This excessive focus on processes of so-called self-organization ignores the presence of contextual factors, which, as noted by Cohen & Stewart (1994), often play a fundamental role in many emergent situations. One of their more striking examples is that of frog gastrulation. When we think of developmental processes, we think of DNA providing a blueprint that describes how all of the varied cellular components are to be differentiated and organized into a complete living organism. In the case of frog gastrulation, however, it is the presence of specific features within the environment that determine whether or not this process is successful. In order for a developing frog embryo to properly gastrulate, that is, to properly fold itself so as to create the gastrointestinal tract, it must exist within a fluid environment having just the right current flow in order to curl the embryo onto itself, thereby forming the tube. If the current is not present, the tube does not form and gastrulation does not occur. In effect, the DNA anticipates the presence of certain external factors in order to carry out its program. Thus in a great many cases emergence is a process that must occur within a particular context, and a knowledge of that context is necessary to understand emergence.
In fact, there is no single process by means of which emergence occurs. One cannot talk about emergence in isolation from contextual factors, whether at higher, lower or similar spatio-temporal scales. For that reason archetypal dynamics emphasizes the notion of an emergent situation, which includes emergents and their context, as opposed to emergence per se, which suggests an emphasis upon intrinsic factors alone, the latter being the typical connotations of the notion of self-organization.
Horizontal emergence
The preceding examples have all involved ideas of vertical emergence, that is, emergence across spatio-temporal scales, although it should be apparent by now that the processes that give rise to such emergence are quite varied, and in general do not reside wholly within the precursor system. There is another very large class of emergent phenomena that is little recognized and that involves horizontal emergence. Horizontal emergence is most typical within the social sciences. In horizontal emergence, the emergent phenomena arise out of and reside at the same ontological level as the precursor phenomena. A familiar example of horizontal emergence is the emergence of culture. The media are awash with images drawn from youth subcultures, which tend to change with each generation. A few individuals within the current cultural milieu develop attitudes and behaviors which begin to distinguish them from others within the larger culture. Over time, others become aware of these new attitudes and behaviors, and if they resonate sufficiently with their psyches they may begin to adopt some of these for their own. In general as they begin to further delineate these differences, the larger culture reacts to these through sanctions prohibitions or sometimes withdrawal or even rejection. These responses serve to further distinguish the new from the old. If enough people adopt the trends of this emerging subculture it may become self-sustaining. Frequently though it will fade away to be replaced by another candidate. If the new culture does persist, it may give rise to a new social unit. It is important, however, to note that both new and old culture exist on the same spatio-temporal scales and are supported by the same kinds of people. It is also important to note that these cultures may become so distinguished as to be completely foreign to one another. Without shared frames of meaning through which to interpret attitudes and actions, misunderstanding and conflict are quite likely.
Another example in which meaning plays a profound role is the diversification of languages. Each language provides a means of communicating meaning between people. In order to use a language effectively, it is usually insufficient to merely learn the rules of grammar and some vocabulary. Anyone who has used those handy travel guides has learned this lesson first hand. In order to communicate effectively one must adopt or at least share in the frame of meaning that is ascribed to words and phrases and sentence constructions within the language. Languages organize thought into very different patterns, with very different emphasis placed on linguistic components. That is why the learning of language within naturalistic settings proceeds more effectively than rote learning from a text. Different languages constitute distinct semantic universes. Nevertheless, new languages do not arise de novo but rather evolve over time from earlier languages. As each language comes to distinguish itself as a unique entity it emerges from the preexisting linguistic context. Again all of this takes place at similar spatio-temporal scales.
Other examples of horizontal emergence include speciation and the evolution of social roles, both of which clearly depend upon contextual factors. The phenomenon of horizontal emergence is not limited to the social sciences. For example, quarks and gluons occupy more or less the same spatio-temporal scales as hadrons, though they differ markedly in character and physical properties. Nevertheless, it is out of the interactions among quarks and gluons that hadrons emerge.
The study of horizontal emergence is particularly salient for the study of emergence more generally because it illustrates clearly how the distinct phenomena of a horizontally emergent situation require distinct frames of meaning for their understanding. Just as distinct frames of meaning are required to navigate among different cultures or to utilize different languages, so too distinct frames of meaning are required to talk about neurons and brain and mental phenomena, or about DNA and species, or about cells and organisms, or about fundamental particles and observable matter. Indeed, in every case of emergence, the emergents are distinguished by frames of reference, which provide meaningful answers to the six basic questions: who, what, where, when, how and why. These frames of reference not only define the emergents, they determine how the environment is to interact with them. They determine both intrinsic (local), and extrinsic (contextual) interactions.
Archetypal dynamics posits the existence of such frames of reference as the fundamental defining characteristic of an emergent situation. Emergent phenomena are not merely distinguished, but are in fact defined by their distinct frames of reference. In a situation of emergence, archetypal dynamics considers both phenomena to be emergents, the situation in which they appear is considered to be an emergent situation, the meaningful or causal influences that one exerts on the other are termed emergence processes, which can therefore be upward, downward, horizontal or reflexive.
The role of meaning in emergence
The applicability of the role of meaning to more general emergent situations can be made clear in the case of a particular example of vertical emergence - the Game of Life, the cellular automaton invented by J. H. Conway, (2001). In order to play the Game of Life, we must understand the following: the basic entities (who) which we can manipulate are the squares on the grid (where), whose colors we can select to be either white or black (what). We are allowed only to determine the initial configuration of colors. The subsequent evolution of the squares occurs in single time steps (when) according to a fixed, deterministic rule (how). If we violate any one of these understandings, we will not have a Game of Life. We may have some other game, or no game at all, but we will not have Life. In addition, in order to determine whether or not we win, we also need to have some notion of the goal of the game (why). In the case of Life, this is arbitrary (though usually specified for any particular play), but it need not be in other emergent situations.
In the search for the presence of non-repeating patterns, Conway and colleagues discovered a wholly remarkable property of Life: the ensuing configurations could serve as ‘OR’ gates, ‘NOR’ gates, ‘NOT’ gates, ‘AND’ gates, and so on. It was then possible to show that much larger patterns of these ‘gates’ could be constructed which could emulate the logical structure of any computer program, and thus emulate the behavior of any computer. It was quite a surprise to realize that the simple Game of Life carried within it the computational ability of a computer.
This computer simulation consists of logic gates and paths (who), arrayed in a grid (where), whose states are given by certain patterns of square colorings (what), which evolve in discrete time steps (when), according to the rules of logic (how), and whose goal is to carry out particular logical computations (why). It is clear that these simulation phenomena arise at a level higher than that of Life, since we are dealing with patterns of squares instead of squares, and obey a different dynamic, logical instead of arithmetical. Moreover, if we are to utilize this simulation effectively, we must impose an initial configuration which encodes both the data and the computation of interest, and whose subsequent evolution must follow logical principles and be interpretable as implementing the computation intended. In addition, we must seek a final configuration which can be meaningfully understood as encoding the result of our computation, and we must have at hand some scheme for carrying out this encoding and decoding of patterns. Life, together with its computational simulations, constitutes an emergent situation, with Life and the simulation being emergents, and the selection process constituting the emergence process whereby we obtain the computational emergent from the Life emergent.
Semantic frames and information
With this example of emergence in the Game of Life in mind, we can go onto to describe the role of Archetypal Dynamics in concerning itself with the epistemological aspects of the emergent situation rather than the emergent processes that give rise to it. It asks, “What fundamentally distinguishes one emergent from another?” In particular, archetypal dynamics focuses upon the role of meaning laden information in distinguishing between emergents. The frame of reference as conceived in archetypal dynamics is termed a semantic frame. It is termed a ‘frame’ because it is an organizing principle, one that parses phenomena into distinct entities, modes of being, modes of behaving, modes of acting and interacting. It is termed ‘semantic’ because it ascribes meaning to all of these in a coherent and consistent manner. The semantic frame shapes and guides our interactions with these phenomena so as to maintain this thread of meaning. The semantic frame provides answers, in whole or in part, to the six basic epistemic questions:
- Who are the relevant entities?
- What are their states, actions, reactions and interactions?
- Where does this all play out?
- When do these events occur?
- How do these events play out? What are the rules governing them?
- Why do these events transpire? What are the goals of entities and their interactions?
The semantic frame is to be understood as a primitive construct, much like a set. A semantic frame both organizes and exerts its influence through the flow of meaning laden information. Information within the context of archetypal dynamics is not to be understood in the sense of Shannon, that is, concerned only with the quantity of information, but rather in its active sense, as ‘the act of informing’ or ‘the state of being informed’, which means ‘to give a special quality or character, to imbue, to instill, to impart by gradual instruction’ (Webster’s Dictionary). Information is to be understood as an efficacious agency that is capable of affecting the characteristics, and ultimately the behavior, of some other. Information may influence the course of events but it does not necessarily cause them. Information possesses content and elicits meaning.
In the ontology of archetypal dynamics, the most basic unit of information is termed an informon. An informon simply refers to any aspect of reality that possesses the capacity to inform. An informon is an aspect of reality that exists prior to any semantic frame. An informon must be attached to, coupled to, or in resonance with, a semantic frame in order to be given form, meaning, and behavior. Consider an analogy. Suppose that one had in their possession two reels of film from two movies. The story and backstory of the movies to which these two reels belong, together with the culture of film, constitute the semantic frames for these reels. They give meaning, coherence and consistency to the reels, and tell us what to do with them. Now suppose that each reel is unrolled and cut up into individual cells, and that the cells are then scattered on the floor. One is now faced with seemingly unrelated bits of experience. Each cell represents a single informon. The semantic frames of the movies allow one to give meaning to the chaos of these single cells, suggesting relationships between them which make it possible to organize the cells into coherent, consistent and meaningful patterns.
Archetypal dynamics posits that information and informons manifest in two distinct ontological forms, active and passive, and two distinct epistemological forms, public and private. The ontological distinction is inspired by the data/program distinction in computer science, and concerns whether the information in question does or does not require the active participation of the informons being related. Passive information is information that informs about properties of informons, or about fixed relationships between informons. Passive information is merely descriptive, like data. Active information, on the other hand, informs about actions and derives from actions. Active information denotes an action of one informon upon another, or an action by or upon some observer or user. Active information instructs, or imposes some choice, or impels some action, like a program.
The epistemological distinction is inspired by the hidden/public distinction in computer science. It concerns whether or not the information in question can impart knowledge to the systems, users and agents coupled to a particular semantic frame. Public information is information that is available to all of the informons comprising a system or user/agent, just like public data, or public programs. Such information is available to any user to guide their subsequent actions. Private information, on the other hand, is information that is not explicitly available to the informons of a system or a user/agent. Nevertheless it is information which influences the behavior of a system or user/agent. This is akin to hidden data like systems files, or hidden programs like systems programs, that are necessary for the operation of the computer system but inaccessible to the user. The public-private distinction is not an artifice, nor does it reduce to a subjective-objective distinction. It has an ontological basis. In physics for example, quark-quark interactions are private, whereas hadron-hadron interactions are public.
Realization, interpretation and representation
Archetypal dynamics posits that real systems relate to semantic frames in one of three primary modes: realization, interpretation, and representation. It is through these modes that one can gain an understanding of the semantic frame in spite of the belief that semantic frames, being conceptual primitives, cannot be fully formalized or defined.
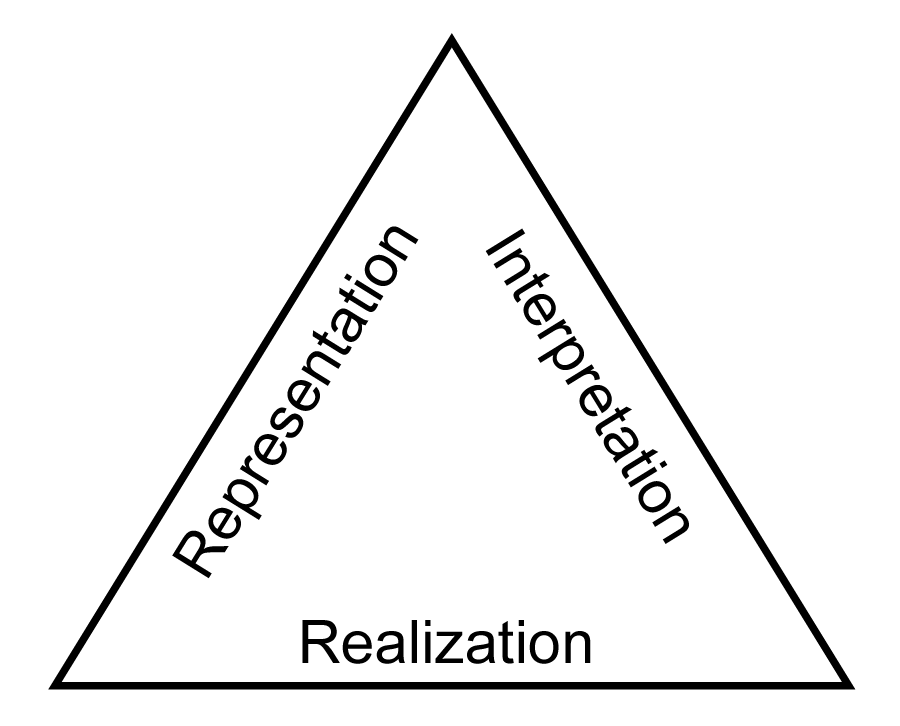
Figure 1 Triadic Composition of Semantic Frames
In addition, archetypal dynamics asserts that real systems may relate to semantic frames in either an active or a passive manner. The passive mode arises from the idea that a semantic frame, as an organizing principle for information, must be about something. It provides definition and description for that something. This does not require the active participation of that which is being defined or described, merely its presence or potentiality, real or imagined. That something for which the semantic frame is about is termed a ‘realization’. A realization may be real, such as an organism, or a physical structure or machine, or it may be ideational, such as a theory or a novel. A realization need not be material but it must be efficacious, that is, it is must be able to make a meaningful difference. A realization of a semantic frame is termed a ‘system’.
Realizations
A semantic frame may have many different realizations, just as a theory in mathematical logic may have many different models (i.e., domains of true interpretation of the logical symbols). Likewise, a system may realize several different semantic frames. If these frames are independent of one another in the sense that one cannot be meaningfully reduced to other, then that system is said to be complex. A semantic frame whose realization is some system is termed an external frame for that system. The external frame tells us how to cut the system into meaningful units, and then provides a meaningful description of how those units relate to one another. The relationship between a system and its external frame is weaker than that between theory and model in formal logic where one can form one-to-one connections between the structures and relations of the model and the formal elements of the theory. Instead, archetypal dynamics suggests that the relationship between system and external frame is more akin to that of a resonance or a coupling, in other words, the ‘goodness of fit’ between a system and its frame reflecting the degree of this resonance. A system may couple to an external frame locally or globally. The stronger and more global the resonance, the more that the semantic frame captures the essence of the system, and the more its dynamics is captured by or influenced by that frame.
Social systems provide a ready example of this. Consider health care systems. In times past, the emphasis in health care systems was placed upon the delivery of health care. One may remember when it was argued that human life possessed inestimable value, and that all possible resources should be devoted to efforts to preserve life. Every effort was directed towards the delivery of care, resulting in large numbers of doctors and nurses, the ready availability of hospital beds and of diagnostic equipment. Delays in the provision of medical care were short. Doctors even delivered care in the home. The semantic frame within which health care personnel and planners operated was one that was health centered. In the past two decades, however, there has been a major shift in health care’s semantic frame. More and more, health has been marginalized as a concern and economics has become the dominant focus. The central concern issue has shifted to how to deliver the least expensive health care, with the result that the standards of health care that are tolerated have progressively declined. There are too few doctors and nurses, too few hospital beds, too long delays in obtaining treatment, premature discharges from hospital, and even though the death rate has not changed, the amount of human suffering has.
Health care delivery is an example of a complex system, one that resonates to varying degrees with several independent semantic frames. The effectiveness of a frame in operating within such a system depends upon the degree of resonance that the system has with the frame. A poor fit results in a very poor interaction with the system. A strong fit results in a very effective interaction. Quite often it is necessary to consider many frames simultaneously when dealing with a system. Attempting to place the onus upon a single frame can lead to inefficiency or to disaster. Based upon the past twenty years experience, it would seem that the over emphasis upon the economic frame has not been beneficial to the delivery of health care to consumers, though it may have been beneficial to governments and insurers.
The Game of Life realizes two external semantic frames: the ‘Life frame’ and the logic gate or ‘computational frame’. Each frame provides a complete description of its particular emergent, sufficient to enable one to interact with that emergent in such a way as to completely explore its dynamics and range of behaviors. These two frames appear to be independent of one another, suggesting that Life fits the criterion for being complex. Moreover the Game of Life couples globally to the Life frame, but only locally to the logic gate frame.
Interpretations
The active mode draws again on inspiration from computer science. A user sits before a computer with some particular problem in mind. That problem will be formulated in terms of data and a program that can be implemented by the computer to provide a solution to the problem. The translation of the original problem into a form that the computer can manipulate requires a semantic frame governing the nature, structure and functionality of a computer, together with an understanding of how to translate real world problems into entities within that semantic frame. The user must interpret the problem through the semantic frame into something accessible to the computer. Based upon this idea, the active mode of a system relating to a semantic frame is termed ‘interpretation’.
Interpretation is an active process, and involves an active relationship with the semantic frame that underlies it. Interpretation gives rise to action, and the actors that implement the action may reside within or outside some system. Those that reside within the system are termed ‘agents’, while those residing outside the system are termed ‘users’. In both cases, the agent/user is the instrument of interpretation, and the results of its interpretation are then manifest through some action either within or upon the system, thereby realizing the interpretation. The semantic frame that a user/agent interprets is termed an internal frame for the user/agent. It is essential to keep in mind that the external frame for a user/agent need not be the same as its internal frame. Indeed, one might argue that it is the existence of separate internal and external frames that defines an entity as a potential user/agent and not merely a system.
Meaning, according to the archetypal dynamics viewpoint, is intimately related to action. Meaning, as a dynamical influence, holds little relevance in situations in which there is no choice, such as in strict deterministic systems or strict stochastic systems. It is only in situations in which a choice must be made that the meanings of individual events and choices become important. Since archetypal dynamics is concerned with meaning laden information, it acquires utility only in situations in which choice plays an important role in the dynamics of systems, which is why the underlying dynamics of archetypal dynamics is based upon the idea of a combinatorial game (see, Berlekamp, et al., 1982; Nowakowski, 1996; 2002.)
Representations
Archetypal dynamics makes a clear distinction between realizations and interpretations of semantic frames, and their representations. A ‘representation’ is understood to be a particular situation, involving a representational frame which serves as a kind of universal object, whose realizations may be associated in some manner with the realizations and interpretations of some other semantic frame. The representation is not itself a realization or interpretation. Realizations and interpretations are ontological constructs, whereas representations are epistemological constructs like symbols and signs. A representation is a symbolic picture, a caricature, a cartoon, to help us to understand the reality. Representations are essential to the pursuit of any formal study, but they are not realizations or interpretations of the semantic frame under consideration, and they must be carefully interpreted in order that a useful understanding of the semantic frame is obtained and paradoxes avoided.
Tapestries and coherence
Archetypal dynamics stipulates that semantic frames act through the organization of informons into meaningful patterns and interactions. A semantic frame thus establishes meaning laden relations among a collection of informons. Semantic frames provide the glue that allows informons to cohere to one another. Although semantic frames may not be directly formalizable, the existence of coherence and of coherent relations between informons provides an opportunity to formalize one aspect of semantic frames.
Coherence may be defined as: 1) holding or sticking together, 2) marked by logical consistency or as congruous, logically connected, or understandable. It is important to also notice that Archetypal dynamics broadens the notion of coherence further to mean ‘meaningfully related’. Two informons are said to be coherent provided that there is a semantic frame that provides a meaningful relationship between them. In the absence of such a meaningful relationship, two informons are said to be incoherent or to be randomly or noisily related. Noise consists of informons that are incoherent or noisily related to one another.
Consistency is often associated with coherence, but archetypal dynamics treats the term consistent in a strictly logical sense in order to indicate that the coherent relations also preserve some kind of logical truth or valuation. Coherence is a local notion, whereas consistency is a global notion.
Coherence, in as much as it provides a link between informons, permits a natural and simple formal representation through the means of the mathematical construct of a graph. The natural representational structure for archetypal dynamics is a particular form of a graph termed a tapestry, (defined in formal notation in Appendices II and III below). The tapestry structure assigns a specific meaning to specific relationships, expressing all of those relationships that are understandable, and therefore all of those relationships that are coherent. The focus upon coherence enables us to construct formal representations of the meaningful linkages imposed upon informons by semantic frames without explicitly defining notions of meaning and of semantics. This avoids many of the pitfalls that have plagued other attempts to express semantic relationships, such as Montague semantics (Thomason, 1970) , Situation Theory (Barwise & Perry, 1983), modal logic (Blackburn, et al., 2001) and circular logic (Barwise & Moss, 1996). These approaches in general posit a universe of meaning that is complete and fixed. Archetypal dynamics, on the other hand, views meaning as ongoing process arising from interpretation. Because meaning in archetypal dynamics involves context, it is always in a state of flux, incomplete and changeable. Meaning is a local construct, requiring an interplay between semantic frame, agent/user, system and context.
Loci and nexi
An informon is a minimal instance that is capable of serving as a unit of information, whether it be in a realization or an interpretation. Informons acquire meaning through interpretation via some semantic frame, and so they form the substrate upon which a semantic frame acts. Informons are organized through coherent linkages, which themselves acquire meaning via the semantic frame. These linkages, being meaningful in their own right, are also conveyors of information, and thus are themselves informons. Informons therefore divide into two fundamental classes depending upon the role that they play. When an informon serves as a dependent element of some coherent relationship, it is termed a ‘locus’. When an informon serves as a defining instance of some meaningful relationship it is termed a ‘nexus’. It is important to note that such distinctions are context dependent, and that a particular informon may serve as both locus and nexus in different relationships.
Traditionally, relations among elements are treated as if imposed from some divine vantage point, being absolute and eternal. Archetypal dynamics, however, views relations as simply a particular class of informon, and therefore relations have a local, transitory quality, arising from within a realization or interpretation rather than being imposed upon it from the outside. These relations may arise out of dynamical interactions that exist intrinsically, independent of any particular semantic frame, or they may arise through the influence of the semantic frame for which they serve as either realization or interpretation. Relations are thus given a dynamic quality in archetypal dynamics, and this inspires the somewhat unusual approach to representing relations by means of tapestries. This dual nature has a counterpart in computer science as previously mentioned. A computer can act upon a file in one of two distinct ways. It may treat it as data, in which case it can act to alter the structure of the file and thereby the information contained within it. It may treat it as a program, in which case it will interpret the structure of the file as being a set of instructions to be carried out. A compiler serves a dual role, treating as data files those files that actually contain programs to be carried out by another computer. Thus files can play either role, or sometimes both, depending upon the specific context. This duality of role is extended to the notion of informon in an analogous manner.
If this approach to relations still seems bizarre and without merit, consider that quantum mechanics suggests that at the smallest scales such fundamental relations as space and time no longer have the same meaning they have classically. For example, the Heisenberg Uncertainty relation suggest that the measurement of one relation may render another relation immeasurable, and so, to that extent at least, it no longer exists. Furthermore, social roles come and go, especially those that depend upon the vagaries of technology. The context defines what relations can arise and whether or not they in fact exist. There is a pernicious tendency to reify and immortalize relations which obscures their ephemeral and parochial nature. However, archetypal dynamics accepts the processual and open-ended nature of relations as a fundamental attribute of all informons, whether locus or nexus.
Loci and nexi, being informons, come in four flavours: active/passive versus public/private. Epistemological constraints force the following restrictions on locus-nexus relationships. A public nexus relates public loci. A private nexus relates loci, at least one of which must be private. A nexus, whether active or passive, will form connections between either all active loci, or all passive loci, but will not mix types.
As a final note on informons, they are to be understood as epistemic units that make no reference to whatever it is that underlies them or gives them existence. Entities, on the other hand, refer to those ontological constructs that serve as generators of informons. This distinction becomes relevant when discussing the more detailed dynamics of tapestries, a subject that will be left for later papers.
General tapestries
The preceding sections have introduced the main philosophical ideas underlying archetypal dynamics and so the focus now turns toward the main formal representational structure pertaining to emergence: the tapestry. The general tapestry serves as a representation for realizations of semantic frames. A generalization of the tapestry concept, the formal tapestry, serves as a representation of interpretations of a semantic frame. A variant of a tapestry, the constructor, serves as the basic element of tapestry dynamics, enabling the definition of the weaving process, which is carried out in the manner of a combinatorial game (these latter aspects are described in Sulis, in preparation 1 and 2). Here the focus will be on general tapestries and the notion of H-complexity. As formal representations of informons and their coherent linkages, the utility of tapestries arises from both their descriptive power and their links to semantics, modal logic, graph theory, group theory, category theory, knot theory, and combinatorial game theory, thus providing access to a large body of mathematical ideas and results.
The formal definition of a tapestry is given in Appendix II. Essentially a tapestry consists of a pair of graphs. One graph consists of struts that convey membership in a particular instance of a coherence relation. The other graph consists of relators that convey the particulars of dependencies which express a coherence relation. Informons that serve as vertices of relators are called ‘loci’. Informons that serve as initial vertices of struts are termed ‘nexi’. A nexus denotes an informon that determines a particular relation among a collection of loci. This is consistent with the view that relations, being conveyers of information, must themselves be informons, but recognizes the distinction between being a conveyer of a relation and being an element that is related within a relation.
A given informon may therefore be either a locus, a nexus, or both, depending upon its connectivity. The labels tie together struts and their associated relators so as to ensure semantic consistency, and can serve as linkages between meaning laden elements in on system and those in another. Although this will not be explored further here, it is possible to assign labels that are derived from some other mathematical object, a group for example, with the relation thereby carrying over some of the group theoretic information. For instance, such might be true if the relation conveyed a distance relation. Note that multiple nexi may define relations on a single label a, and a given nexus may define relations on multiple labels.
In most formal models of relations there is a distinction between the relation itself and the objects that are related. In logic, this distinction gives rise to various orders of logic, with each order being able to express concepts related to all orders below it but none above it. These order distinctions enable the expression of many subtleties but also induce many limitations. The tapestry approach enables relations of multiple orders to be expressed within a common framework. This gives the tapestry structure considerable expressive power.
In general, the collections of informons, struts, relators, and labels will each be divided into four distinguished subsets corresponding to the active/passive, public/private distinctions and which must adhere to certain coherence conventions which will not be discussed here. The interested reader can examine these elsewhere (Sulis, 2002; Sulis, submitted; Sulis, in preparation 1 and 2).
H-complex tapestries
We need to recall that the stated, ultimate goal of archetypal dynamics is to shed light on the phenomena of emergence. Although, we’ve been describing different forms of emergence, particularly the distinction between vertical, horizontal, and reflexive emergence, in all of the cases emergents are distinguished through distinct, independent semantic frames which provide answers to the fundamental epistemological questions of who, what, when, where, how, and why that define each emergent. The presence of distinct emergents thus implies that a system admits description through distinct, independent semantic frames, which in turn suggests our definition of a complex system which is capable of supporting emergent behavior, as a system able to realize multiple independent semantic frames.
Horizontal emergence offers itself perhaps as the easiest to grasp through this formalism of semantic frames. One reason is that the phenomena of horizontal emergence appear at the same spatio-temporal scales, and generally involve entities of similar kind. This enables us to avoid an infinite regress when engaging in a discussion of the concept of an entity. Accordingly, let’s apply the notion of the realization of multiple independent semantic frames to horizontal emergence: if a system realizes multiple independent semantic frames all at the same spatio-temporal scales, indeed, at the same scales whatever, this suggests that the basic informons of the system admit distinct interpretations through multiple independent semantic frames. This further means that these informons can be represented by distinct tapestries, one for each of these independent semantic frames.
Although not absolutely necessary, since this system could represent a special case, it nevertheless seems a reasonable assumption that the tapestries expressing emergent systems are non-isomorphic. The reason is that it is commonly understood within the mathematical community that for two mathematical entities to be isomorphic means that they differ, essentially, only in the names given to the entities involved, and since names can be chosen arbitrarily, this means they represent the same thing. If it was the case that two semantic frames were never to give rise to non-isomorphic tapestries then, at least within the archetypal dynamics framework, we would have no means of distinguishing between them, and so for all intents and purposes they would appear as if they were the same. It appears sensible consequently to assume that we are dealing, in the case of horizontal emergence, with a situation in which these frames are distinguishable and thus representable by non-isomorphic tapestries.
Since we are considering horizontal emergence and the same basic collection of informons and linking relations, this suggests that we have one underlying tapestry which represents the informons and their linking relations but somehow we have this single tapestry representing distinct semantic frames. That would imply one tapestry appearing in non-isomorphic forms. That would, however, be nonsensical. But recall that the role of a semantic frame is to give meaning to entities and relations, and that in the tapestry construction specific meaning is conveyed through the use of the meaning labels. The struts and relators provide the structural information but the meaning information lies in the labels. So this opens up one possibility, namely, whether it is possible to start with the same basic tapestry structure, including the same basic collection of struts and relators, and on this structure impose distinct labelings which are consistent with this structure and yet result in the creation of distinct non-isomorphic tapestries.
From a mathematical standpoint this may be formulated as follows. Given some tapestry T, we may strip away all of the labels leaving a bare tapestry, B(T). We now ask whether it is possible to find a new tapestry T', which is non-isomorphic to T yet such that B(T) = B(T'). A tapestry with this property is said to be H-complex. A tapestry for which no such non-isomorphic relabeling exists is termed H-simple.
This turns out to be a good definition and there are already a number of basic results concerning the existence and nonexistence of H-complex and H-simple tapestries (Sulis, submitted). Although there are an infinite number of H-simple tapestries, the conditions for their existence are quite strict and limiting. As a consequence, it is far more likely that any naturally occurring tapestry will be H-complex, and thus the conditions for the possible occurrence of horizontal emergence should be ubiquitous.
As an aside, one should note that the definition of H-complexity as stated above is actually too general, resulting in a rather narrow collection of H-simple tapestries. To correct this, it is necessary to introduce the idea of relative H-complexity. The basic idea is to associate the general tapestry with a formal tapestry that serves as a system of constraints, and to require that any relabeling of the general tapestry be consistent with this formal tapestry. This is akin to requiring that any relabeling of the tapestry satisfy a collection of symmetries. This issue is discussed further in Sulis (submitted).
Conclusion: A scenario for horizontal emergence
The mere statement of a mathematical condition for H-complexity does not at all prove that it has anything to do with horizontal emergence as it appears in real systems. So let us consider one possible scenario within which horizontal emergence might appear. Suppose that we have a large population of agents acting within a system. Each agent will interpret the events surrounding it according to some internal semantic frame and so each agent could, in principle, construct a tapestry which represents these events with meanings associated to its semantic frame. In a homogeneous world, all of the agents would be acting under the influence of the same semantic frame, and would be witness to more or less the same set of events, at least in principle. Even if these agents did not have access to all of the information available, they would have access to local information and one would at the very least expect that those events that were perceived in common by groups of agents would give rise to one and the same tapestry.
Although it is not a prerequisite of the theory, let us assume that the semantic frame of the agents has its origin within the physical-dynamical properties of each agent. One mechanism through which such a physical-semantic coupling might come about is through TIGoRS (Transient Induced Global Response Stabilization described in (Sulis, 1995, 2000, 2002). In TIGoRS, a non-autonomous dynamical system exhibits a stable dynamical response to specific patterned inputs. Depending upon the particular dynamical system, these inputs may have patterns that are structured or unstructured (random). Thus each such dynamical system parses its experience uniquely into patterns that stabilize and patterns that do not. It has been shown that such a parsing enables the dynamical system to function as a grammatical rewriting system. In other words, the system treats each pattern as if it were a unit of information and generates a new unit of information expressed behaviorally and linked specifically to that unit which it had received.
Even in the absence of any intrinsic property of adaptation one can still assume that agents are subject to fluctuations in structure and dynamics. It is plausible that at some time, agents are created that possess an intrinsic semantic frame which differs in essential ways from that of the larger population. In many cases these will lead to only transient aberrations since these frames will not couple in any substantive way with the larger system. But if the system is such that its tapestry is H-complex, then it possesses an inherent ambiguity. This means that over time it is possible that agents could be created whose semantic frames generate tapestries that resonate with the system, meaning that they are compatible with the bare tapestry of the system and yet are no longer isomorphic with that generated by the population as a whole. One expects a stable resonance to be created in such a circumstance.
Now suppose over time that more such agents are created, whether through reproduction mechanisms or adaptation or some other selection mechanism. It is natural that such agents, sharing a common semantic frame, and perceiving the system through a common tapestry, come over time to interact more with one another than with the other agents. It is possible that the other agents, no longer sharing a common frame of reference, might begin to distance themselves from these agents. Either way it is possible that there come to exist two distinct sub-collections of agents with distinct semantic frames co-existing within the same system. It is possible that over further time, structural and dynamical changes may cause alterations in patterns of behavior that generate informons that are no longer compatible with a shared underlying bare tapestry, but generate wholly distinct tapestries. In this event, two entirely distinct systems are created, that is, emergence.
The scenario presented here is purely a thought experiment and meant solely to suggest that the H-complexity notion shows how horizontal emergence may take place. It is not meant to suggest that this is how it happens in general, or even at all. Hopefully, the reader will think it is sufficiently plausible to warrant further investigation.
Appendix I: Diagonalization and Russell’s paradox
To illustrate Goldstein’s Diagonalization, one of the scenarios underlying his idea of a self-transcending construction, assume that one has constructed the collection of all maps from the natural numbers to itself. Suppose further that one can assign a natural number to each map. Denote the map corresponding to n by fn. Now create an array aij, indexed by the natural numbers, by setting aij= fi(j). Now define a new map from the natural numbers to itself by defining g(k) = fk(k)-1 if fk(k) ≠ 0, and 1 otherwise. Clearly g cannot be one of the original functions because if it could be assigned a number n then we would have either g(n) = fn(n) = fn(n)-1 or 1 = g(n) = fn(n) = 0. Thus, from the natural numbers one creates a new collection that transcends the original in size, or in other words, has transcended the set from which it emerged.
Another transcendental construction gives rise to Russell’s Paradox. A set is just a collection of elements. From any set one can form a subset by simply taking some elements from the set and creating out of them a new set. It is easy to see that one can create sets that do not contain themselves as members. For example the set {i, 2} does not contain {1, 2} as a member as it contains only 1 and 2. So let us now construct a new object X which consists of all sets that do not contain themselves as members. We have just demonstrated that such an object must be non-empty, so it appears to be a good object. Suppose that it is a set. If X ∉ X, then X is a set which does not contain itself as a member, so by the definition, X ∈ X. If X ∈ X, then again by the definition, X must be a set which does not contain itself as a member, so X ∉ X. In both cases we achieve a contradiction, which forces [X] to be a new object which transcends the concept of set. Russell called such objects classes.
Appendix II: Tapestries
Here I present some of the technical details concerning tapestries and the concept of H-complexity.
Definition: A graph is a pair (V, E) where V is a set of vertices and E is a collection of two element subsets of V, that is, E consists of subsets of the form {a,b} where a, b ∈ V. A graph is depicted as a diagram in which each vertex is represented as a point and each edge is represented as a line joining the points corresponding to its vertices.
Definition: A directed graph (digraph) is a pair (V, E) where V is a set of vertices and E ⊂ V x V is a set of edges. Each edge is represented as an ordered pair (a, b). There are two associated maps i: E → V defined by i(e) = i(a, b) = a, which assigns the initial vertex of e and t: E → V defined by t(e) = t(a, b) = b, which assigns the terminal vertex of e. A directed graph also has a diagram in which the line representing an edge is replaced by an arrow pointing from initial to terminal vertex.
Definition: A labeled graph (digraph) is a triple (V, E, L) where (V, E) is a graph (digraph), L is a set of labels, and there is a mapping l: E → L which provides a labeling of each edge.
Definition: A multigraph (directed multigraph) is a graph (digraph) in which more than one edge may link any two vertices. U sually the edge s in a multigraph are labeled so as to distinguish them but this is not necessary in general.
Definition: A tapestry is a 4-tuple (I, S, R, Ω) where:
- I is a collection of informons;
- S is a collection of struts;
- R is a collection of relators;
- Ω is a collection of meaning labels;
- TS = (I, S)is an Ω-labeled, directed multigraph;
- TR = (I, R)is an Ω-labeled, directed multigraph;
- Given any a, b in I and label α, there exist at most two α-labeled relators, r, r' in R with r: a → ab and/or r': b → αa;
- For any a, b in I and any α-labeled relator r in R with r: a → αb, there exists exactly one n in I and α struts s, s' in S such that s: n → αa and s': n → αb;
- Every n in I is either a vertex of some r in R or an initial vertex of some s in S, or both. That is, there are no isolated vertices.
A vertex of a relator is termed a locus. An initial vertex of a strut is termed a nexus. The terminal vertex of a strut may be a locus or a nexus. The set of loci is denoted L. The set of nexi is denoted N. Thus I = L ∪ N. A vertex is locus or nexus depending upon context.
A tapestry is graphical if (I, R, Ω) is a directed graph, and proper if each nexus is associated with exactly one label.
For simplicity we may denote an edge e: x → αy by ea(x,y).
A given informon may therefore be either a locus, a neuxs, or both, depending upon its connectivity and context. A locus represents an instance of a relation while a nexus defines a relation. The struts associated with a nexus identify those loci that participate in the relation. The labels tie together struts and their associated relators so as to ensure semantic consistency. N ote that multiple nexi may define relations on a single label a, and a given nexus may define relations on multiple labels.
In general, the sets I, S, R, Ω will each be divided into four distinguished subsets corresponding to the active/passive, public/private distinctions and the coherence conventions must be adhered to (see Sulis, 2002).
Appendix III: H-complex tapestries
Definition 1: Let T = (L, S, R, Ω) be a tapestry. The bare tapestry corresponding to T is the triple B(T) = (L, S, R) formed by stripping off all of the labels. The tapestry skeleton of T is the pair U(T) = (L, S ∪ R) which is the bare tapestry with the distinctions between struts and relators eliminated.
Definition: Let G = (V, E) and G' = (V', E') be multigraphs. A multigraph morphism f: G → G' is a pair (a, b) where a: V → V', b: E → E' are maps such that if e = {x, y} and e' = {x', y'} and b(e) = e', then x' = a(x) and y = a(y). A similar definition holds for directed multigraphs. In the case of graphs and digraphs one can eliminate the map b since there is at most only one edge per vertex pair in the case of a graph, and the ordering for a digraph distinguishes edges.
Definition: Let T = (I, S, R, Ω) and T' = (I', S', R', Ω') be tapestries with labelings l, l' respectively. Then a tapestry morphism is a map f: T → T' such that f = (a, b, c, d) where a: I → I', b: S → S', c: R → R', d: Ω → Ω' and:
- (a, b): (I, S) → (I', S') is a directed multigraph morphism,
- (a, c): (I, R) → (I', R) is a directed multigraph morphism,
- d is a set morphism such that dl = l'(a, b) and dl = l'(a, c).
A tapestry morphism is an isomorphism if all of the associated maps are isomorphisms.
Definition: Let T = (I, S, R, Ω) be a tapestry with labeling l. Then a tapestry T' = (I, S, R, Ω') with labeling l'is termed a relabeling of T if l ≠ l'.
Definition: A tapestry T is said to be H-complex if there exists a tapestry T' which is non isomorphic with T such that B(T) = B(T'). A tapestry T is said to be H- simple if all tapestries having the same bare tapestry as T are isomorphic to T. Mathematically, a tapestry is H- complex provided that its bare tapestry admits multiple non-isomorphic labelings, and is simple otherwise.
References
Anderson, P. B., Emmeche, C., Finnemann, N. O. and Christiansen, P. V. (1995). Downward causation: Minds, bodies and matter, Aarhus, Denmark: Aarhus University Press.
Barwise, J. and Perry, J. (1983). Situations and attitudes, Cambridge, MA: MIT Press.
Barwise, J. and Moss, L. (1996). Vicious circles, Stanford, CA: CSLI Publications.
Berlekamp, E., Conway, J. H. and Guy, R. (1982). Winning ways for your mathematical plays, NY: Academic Press.
Blackburn, P., de Rijke, M. and Venema, P. (2001). Modal logic, New York, NY: Cambridge University Press.
Cohen, J. and Stewart, I. (1994). The collapse of chaos, New York, NY: Viking Press.
Conway, J. (2001). On numbers and games, 2nd edition, A. K. Peters (ed.), Natick, MA: A. K. Peters.
DeLaszlo, V. (1959). The basic writings of C. G. Jung, New York, NY: Modern Library.
Forrest, S. (1990). “Emergent computation,” Physica D, 42: 1-11.
Goldstein, J. (1999). “Emergence as a construct: History and issues,” Emergence: Complexity Issues in Organization and Management, 1(1): 49-62.
Goldstein, J. (2003). “The construction of emergent order, or, how to resist the temptation of hylozoism,” Nonlinear Dynamics, Psychology, and Life Sciences, 7(4): 295-314.
Nowakowski, R. (1996). Games of no chance, New York, NY: Cambridge University Press.
Nowakowski, R. (2002). More games of no chance, New York, NY: Cambridge University Press.
Sulis, W. (1995). “Driven cellular automata” in D. Stein and L. Nadel (eds.), 1993 Lectures in complex systems, Reading, MA: Addison-Wesley.
Sulis, W. (2000). “The neural basis of mental representations: Nonlinear perspectives” in I. Trofimova and W. Sulis (eds.), Nonlinear Dynamics in the Life and Social Sciences, Brussells: IOS Press, pp. 98-132.
Sulis, W. (2002). “Archetypal dynamics: An approach to the study of emergence” in J. Nation, I. Trofimova, J. Rand and W. Sulis (eds.), Formal descriptions of developing systems, NATO science Series II/121, Kluwer, pp. 185-228.
Sulis, W.(Submitted). “Archetypal dynamics 1: Horizontal emergence,” submitted to Nonlinear Dynamics, Psychology, and Life Sciences.
Sulis, W. (In preparation 1). “Archetypal dynamics 2: The reality game.”
Sulis, W. (In preparation 2). “Archetypal dynamics 3 : Vertical emergence.”
Thomason, R. (1970). Formal philosophy: Selected papers of Richard Montague, New Haven: Yale University Press.